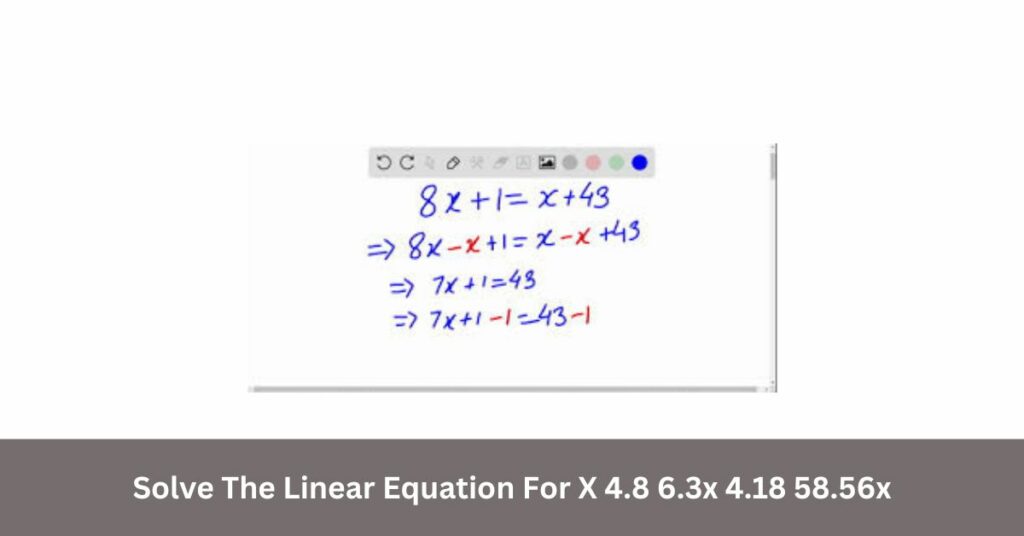
To Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x combine all constant terms (4.8 + 4.18) and terms with x (6.3x + 58.56x). This results in a simplified equation: 64.86x + 8.98. Any value of x that makes this equation true is the solution.
we’ll discuss how to solve the linear equation For X 4.8 6.3x 4.18 58.56x, breaking it down into simple steps. Learn easy techniques to find the solution and understand the process effortlessly.
What Are Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x?
To solve the linear equation For X 4.8 6.3x 4.18 58.56x, we aim to find the value of \(x\) that makes both sides of the equation equal. By isolating (x), we can simplify the equation and determine its solution.
This process involves combining like terms and performing arithmetic operations to ensure a clear understanding of the relationship between the variables. Ultimately, solving such equations enables us to analyze real-world problems involving linear relationships and make informed decisions based on the calculated values.
Explore Linear Equations – Simplifying Math With Straight Lines!
Linear equations are mathematical expressions that describe the relationship between variables in a straight line. They typically take the form (ax + b = c), where (a), (b), and (c) are constants, and (x) is the variable we’re solving for.
These equations are fundamental in algebra and are used to solve various real-life problems, such as calculating costs, determining rates of change, or predicting future outcomes. By understanding linear equations, we can analyze and solve problems involving proportional relationships straightforwardly.
When Do You Need To Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x? – Explore It!
You may need to Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x whenever you encounter situations involving proportional relationships or unknown values. This could include scenarios such as calculating costs and determining rates of change.
Predicting future outcomes in various fields like finance, physics, engineering, and everyday life problems. By solving such equations, you can find the value of (x) and understand how different variables linearly interact with each other.
Why Should You Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x? – Must Read!
1. Real-world Applications:
Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x helps in everyday situations like managing finances, determining distances in travel, or analyzing changes in quantities over time. By finding the value of X, we can accurately predict outcomes and make informed decisions in various real-life scenarios.
2. Problem-solving Skills:
Mastering linear equations enhances problem-solving abilities significantly. By breaking down complex situations into simpler mathematical expressions, individuals develop critical thinking and analytical skills. These skills are invaluable in navigating various challenges in both academic and real-world contexts.
3. Understanding Relationships:
Solving equations to find the value of x provides insights into how different variables interact linearly. This understanding is crucial for making predictions and decisions based on data analysis. It allows individuals to grasp the underlying relationships between different factors and make informed choices.
4. Academic Success:
Proficiency in linear equations is essential for success in mathematics education. It forms a solid foundation for tackling more advanced topics in algebra, calculus, and other mathematical disciplines. By mastering linear equations, students build confidence and competence in their mathematical abilities.
5. Career Advancement:
The ability to solve linear equations is highly sought after in various fields such as finance, engineering, science, and economics. Proficiency in this area opens doors to career opportunities and advancement. Employers value individuals who can analyze data, solve problems, and make informed decisions using mathematical principles.
Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x – Step-by-Step Guide to Finding x!
- Identify Like Terms: Begin by identifying the terms containing x, which are 6.3x and 58.56x. Also, identify the constants, which are 4.8 and 4.18.
- Combine Like Terms: Add or subtract the coefficients of like terms. In this case, add 6.3x and 58.56x to get 64.86x. Similarly, add 4.8 and 4.18 to get 8.98.
- Isolate the Variable: To isolate x, move all terms with x to one side of the equation and constants to the other side. Subtract 64.86x from both sides to get 8.98 = 58.56x – 6.3x.
- Combine Coefficients: Simplify the equation by subtracting 6.3x from 58.56x to get 52.26x. Now the equation becomes 8.98 = 52.26x.
- Solve for x: To find the value of x, divide both sides by 52.26. x = 8.9852.26 0.172.
- Verify the Solution: Substitute the value of x back into the original equation to ensure it satisfies the equation.
- Finalize: The solution for x is approximately 0.172.
By following these steps, you can effectively Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x and find the value of x.
Common Mistakes To Avoid When Solving Linear Equations:
When solving linear equations, it’s crucial to watch out for common mistakes that can lead to incorrect answers. One common error is forgetting to perform the same operation on both sides of the equation, which can throw off the balance and result in an inaccurate solution.
Another mistake is misidentifying like terms and incorrectly combining them, leading to errors in simplification. Additionally, overlooking signs or arithmetic operations can easily result in computational errors, impacting the final solution.
It’s also important to be mindful of the order of operations and follow them carefully to ensure accurate results. By being aware of these common pitfalls and double-checking each step, you can avoid mistakes and arrive at the correct solution when solving linear equations.
Frequently Asked Questions:
1. Why is it important to solve linear equations?
Solving linear equations is crucial for various real-life applications, such as budgeting, calculating distances, determining rates of change, and making predictions. It also helps in developing problem-solving skills and understanding relationships between different variables.
2. Can linear equations be solved using different methods?
Yes, linear equations can be solved using various methods such as substitution, elimination, graphing, and matrices. The choice of method depends on the complexity of the equation and personal preference.
3. Are there any prerequisites for solving linear equations?
It’s helpful to have a basic understanding of algebraic concepts such as variables, constants, coefficients, and arithmetic operations. Familiarity with the order of operations (PEMDAS/BODMAS) and solving simple equations is also beneficial.
4. How can I check if my solution to a linear equation is correct?
You can verify your solution by substituting the value of x back into the original equation and ensuring that both sides of the equation are equal. If the equation holds, then your solution is correct.
Final Words:
Mastering the process of Solve The Linear Equation For X 4.8 6.3x 4.18 58.56x equips you with essential problem-solving skills and opens doors to understanding various real-world scenarios.
Keep practising and applying these techniques to confidently tackle similar equations and excel in mathematics.
Read:
- VenturesGet.net – Essential Tools for Startup Success in 2025!
- Rena Monrovia When You Transport Something By Car …-Essential Tips
- West vs East Match Player Stats- An In-Depth Analysis!
- Igagony -The Secret Way To View Instagram Stories Anonymously
- GMFU Meaning- The Go-To Acronym for Frustration Online!